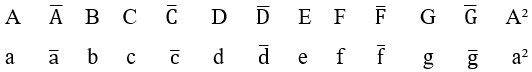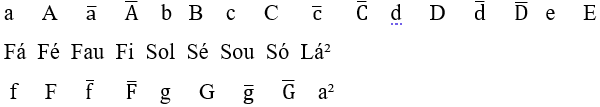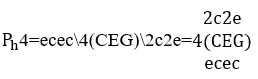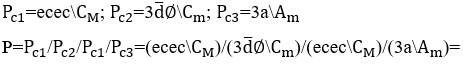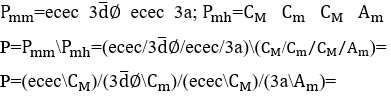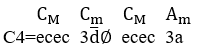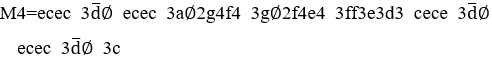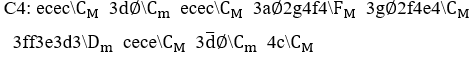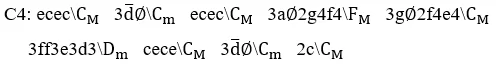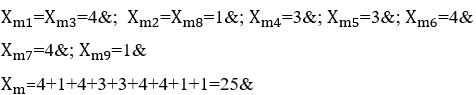ORIGINAL ARTICLE
VIANA, Arnóbio Araújo [1]
VIANA, Arnóbio Araújo. The mathematical structure of music. Revista Científica Multidisciplinar Núcleo do Conhecimento. Year. 07, Ed. 08, Vol. 02, p. 196-220. August 2022. ISSN: 2448-0959, Access link: https://www.nucleodoconhecimento.com.br/mathematical-olympiads/mathematical-structure-of-music, DOI: 10.32749/nucleodoconhecimento.com.br/mathematical-olympiads/mathematical-structure-of-music
ABSTRACT
Taking into account that musical composition is a sound mathematical structure, this article demonstrates the development of the Mathematical Structure of Music, disseminating a more understandable sound language and adapted to the current technology of scientific studies related to the Science of Music. Therefore, the objective of this research was to develop an algebraic language to mathematically structure Music, using only letters, numbers and symbols to write the sounds of musical notes in an alphanumeric way, representing its main sound characteristics, such as: frequency (f) , amplitude (a) and duration time (t), in a single expression to identify a sound wave. The developed language allows a spelling with simple reading and allows the study of musical phenomena in general on a platform with graphic representations in the Cartesian coordinate system of the structures of the melodic groupings, with the musical notes in melody and the harmonic groups with the notes in harmony, thus promoting the Mathematical Structure of Music.
Keywords: Musical Cell, Melody, Harmony, Rhythm.
1. INTRODUCTION
This research was made possible by the development of the Harmonization or H operations and its inverse Melodyation or M, established in the article “the harmonization operation (H) and its inverse melody operation (M)” (VIANA, 2022).
In the aforementioned article, it is defined that the Operation H (\), between sound waves of musical notes, form a harmonic grouping, whose harmony (h) is the sound effect of the result of a combination between them ![]() . In this case, these waves are interconnected simultaneously in space-time, that is, their initial times are equal and their final times are also equal. These waves of equal musical notes form a harmonic grouping of a single sound or a unison harmony
. In this case, these waves are interconnected simultaneously in space-time, that is, their initial times are equal and their final times are also equal. These waves of equal musical notes form a harmonic grouping of a single sound or a unison harmony ![]() (VIANA, 2022).
(VIANA, 2022).
Its inverse Operation M (\), is defined between sound waves of musical notes that form a melodic grouping, whose melody is the sound effect of the result of the arrangement between them ![]() . In this case, these waves are continuously interconnected in space-time, that is, the final time of a wave is equal to the initial time of the next wave and so on, where waves of equal musical notes form a melodic grouping of repeated sounds
. In this case, these waves are continuously interconnected in space-time, that is, the final time of a wave is equal to the initial time of the next wave and so on, where waves of equal musical notes form a melodic grouping of repeated sounds ![]() . In this way, the musical groups formed and organized in space-time will constitute a musical composition, composed of melody, harmony and rhythm (VIANA, 2022).
. In this way, the musical groups formed and organized in space-time will constitute a musical composition, composed of melody, harmony and rhythm (VIANA, 2022).
Therefore, the objective of this research was to develop an algebraic language to mathematically structure Music, using only letters, numbers and symbols to write the sounds of musical notes in an alphanumeric way, representing its main sound characteristics, such as: frequency (f) , amplitude (a) and duration time (t), in a single expression ![]() to identify a sound wave.
to identify a sound wave.
2. REPRESENTATION OF A MUSICAL NOTE
PTo represent the sound of a musical note algebraically in this research, three fundamental characteristics are necessary: the sound frequency (f); the amplitude (a); and the duration time (t), forming the expression “![]() ”, called, in this study, the Musical Cell.
”, called, in this study, the Musical Cell.
This nomenclature was given because it represents any sound or non-sound wave, or even any attribute that composes the structure of a song, with the musical note being the most important sound cell to Music, and the musical pause, the most important non-sound cell to the Music. Music, considered a silent or soundless musical note, where the zero amplitude frequency ![]() is represented by a negated zero
is represented by a negated zero ![]() as your cipher
as your cipher ![]() in this Musical Algebraic Language.
in this Musical Algebraic Language.
2.1 REPRESENTATION OF MUSICAL FREQUENCY
Frequency (f), in general, is the most important characteristic of any vibration and is caused by an earthquake in any medium, which will produce a series of frequencies. When in Operation H natural between them, a harmony is formed, which determines the resulting frequency of this shake ![]() . In addition, the one with the lowest sound or the lowest oscillation between them
. In addition, the one with the lowest sound or the lowest oscillation between them ![]() , is the most important of all, called the Fundamental Frequency
, is the most important of all, called the Fundamental Frequency ![]() , being the characteristic responsible for the sound we hear and that allows us to distinguish a bass or low-frequency sound from a high-pitched or high-frequency sound.
, being the characteristic responsible for the sound we hear and that allows us to distinguish a bass or low-frequency sound from a high-pitched or high-frequency sound.
Represented in Music, currently, based on the work of Guest (2020, p. 33 to 41), by the first seven letters of the Latin Alphabet A, B, C, D, E, F and G, called ciphers, respectively representing the musical notesLá, Si, Dó, Ré, Mi, Fá and Sol, where in capital letters they will indicate the harmonies of instrumental accompaniments or Musical Chords that, in this study, will also represent the musical notes grouped in harmony, result of the Operation ” H” among them ![]() . The lowercase letters will represent the musical notes grouped in melody, the result of Operation “M” between them
. The lowercase letters will represent the musical notes grouped in melody, the result of Operation “M” between them ![]() .
.
The eighth musical note of the alphabet mentioned above is the repetition of the first note ![]() , twice as often, identified by a numeric index two on the upper side of its cipher
, twice as often, identified by a numeric index two on the upper side of its cipher ![]() and, if this index were positioned below
and, if this index were positioned below ![]() , this note would be called the Low Octave, with its frequency halfway down the first note, meaning that its frequencies are decreasing
, this note would be called the Low Octave, with its frequency halfway down the first note, meaning that its frequencies are decreasing ![]() .
.
Therefore, a musical note scale in octave can be represented in parentheses with its indicative index above ![]() or low octave with its indicative index below
or low octave with its indicative index below ![]() .
.
Among the eight main musical notes ![]() , yet, there are five other intermediate notes, currently represented with the symbol of sharp
, yet, there are five other intermediate notes, currently represented with the symbol of sharp ![]() next to its cipher on an increasing scale in Lá
next to its cipher on an increasing scale in Lá ![]() , separated by frequency spaces called a semitone
, separated by frequency spaces called a semitone ![]() .
.
However, in this study, the word “Sharp” is replaced by the letter “u” and its symbol ![]() by a slash over the cipher
by a slash over the cipher ![]() , to provide a monosyllabic or one-syllable name, which can be sung by anyone in the solfeggio study of these musical notes. Thus, the musical note Lá sharp
, to provide a monosyllabic or one-syllable name, which can be sung by anyone in the solfeggio study of these musical notes. Thus, the musical note Lá sharp ![]() is also the note Lau
is also the note Lau ![]() , Dó sharp
, Dó sharp ![]() the note Dou
the note Dou ![]() , Ré sharp
, Ré sharp ![]() the note Reu
the note Reu ![]() , Fá sharp
, Fá sharp ![]() the note Fau
the note Fau ![]() and Sol sharp
and Sol sharp![]() the note Sou
the note Sou ![]() .
.
Yet, too, there are twelve musical notes in between, between the thirteen notes of the crescent semitone scale in Lá ![]() , separated by frequency spaces called a Microtom
, separated by frequency spaces called a Microtom ![]() , used, normally, by musicians from the Eastern Continent, who, in this study, present their names with their figures, originated with the first letter of the note before their position in the ascending scale in Lá
, used, normally, by musicians from the Eastern Continent, who, in this study, present their names with their figures, originated with the first letter of the note before their position in the ascending scale in Lá ![]() , joined to a vowel (a, e, i, o, u), excluding those that repeat the names of existing notes.
, joined to a vowel (a, e, i, o, u), excluding those that repeat the names of existing notes.
For example, the first microtone note “![]() ” between the notes Lá and Lau
” between the notes Lá and Lau ![]() , have your name “Lé”, formed with the letter L of the note A (a), plus the vowel “e” of the sequence “a, (e), i, o, u”, excluding the vowel “a” of the note Lá and its cipher is the same of the note Lá (a), but in capital letter (A) when in melody, as well as the name of the next microtone note “
, have your name “Lé”, formed with the letter L of the note A (a), plus the vowel “e” of the sequence “a, (e), i, o, u”, excluding the vowel “a” of the note Lá and its cipher is the same of the note Lá (a), but in capital letter (A) when in melody, as well as the name of the next microtone note “![]() ” between the notes Lau and Si
” between the notes Lau and Si ![]() , have your name “Li”, formed with the letter L of the note Lau
, have your name “Li”, formed with the letter L of the note Lau ![]() , plus the vowel “i” of the sequence “a, e, (i), o, u”, excluding the vowels “a” and “e” of the notes Lá and Lé, its number being the same as the note Lau
, plus the vowel “i” of the sequence “a, e, (i), o, u”, excluding the vowels “a” and “e” of the notes Lá and Lé, its number being the same as the note Lau ![]() , but in capital letter
, but in capital letter ![]() and, the name of the twelfth musical note
and, the name of the twelfth musical note ![]() between the note Sou and Lá octave
between the note Sou and Lá octave ![]() , it is “Só”
, it is “Só” ![]() , being formed by the letter “S” in Sou and “o” from the vowel sequence “a, e, i, (o), u”, excluding the vowels “a” existing in Sá, “e” existing in Sé, “i” existing in Si, leaving the vowel “o”. Your number is the same as the note Sou
, being formed by the letter “S” in Sou and “o” from the vowel sequence “a, e, i, (o), u”, excluding the vowels “a” existing in Sá, “e” existing in Sé, “i” existing in Si, leaving the vowel “o”. Your number is the same as the note Sou ![]() , but in capital letter
, but in capital letter ![]() . This is how the musical notes microtones were called, below the semitone scale and the microtone scale in Lá.
. This is how the musical notes microtones were called, below the semitone scale and the microtone scale in Lá.
Semitone musical scale in Lá
Lá Lau Si Dó Dou Ré Reu Mi Fá Fau Sol Sou Lá2
Microtone musical scale in Lá
Lá Lé Lau Li Si Sá Dó Dá Dou Dé Ré Rá Reu Ri Mi Má
2.2 TONE  , SEMITONE
, SEMITONE  AND MICROTOM
AND MICROTOM 
Whatever the musical scale, its musical notes are separated in melody by spaces of frequency intervals ![]() , which can present three measurements at different frequencies, called Tone
, which can present three measurements at different frequencies, called Tone ![]() , Semitone
, Semitone ![]() and Microtom
and Microtom ![]() , being the semitone determined through its scale of thirteen musical notes
, being the semitone determined through its scale of thirteen musical notes ![]() ,which is a series of a GP or Geometric Progression, (SILVA, s.d.).
,which is a series of a GP or Geometric Progression, (SILVA, s.d.).
In this case, a Musical GP (MGP), where the thirteenth note is twice the first ![]() . Therefore, one can apply the Nth Term formula of a GP
. Therefore, one can apply the Nth Term formula of a GP ![]() and calculate your reason
and calculate your reason ![]() , where
, where ![]() and
and ![]() , resulting in the interval of a semitone
, resulting in the interval of a semitone ![]() , tone being calculated by the square power of a semitone
, tone being calculated by the square power of a semitone ![]() and the microtone calculated by the square root of a semitone
and the microtone calculated by the square root of a semitone ![]() .
.
These values can also be determined by the general formula of frequency intervals ![]() where “n” equal to one
where “n” equal to one ![]() results in the interval of a semitone
results in the interval of a semitone ![]() “n” equal to two
“n” equal to two ![]() results in the range of a tone
results in the range of a tone ![]() “n” equal to half
“n” equal to half ![]() results in the range of a microtone
results in the range of a microtone ![]() In this way, the frequency of a musical note can be calculated through the formula of the General Term of a GP
In this way, the frequency of a musical note can be calculated through the formula of the General Term of a GP ![]() where the first term
where the first term ![]() is the standard fundamental frequency of the musical note Lá
is the standard fundamental frequency of the musical note Lá ![]() and the second term
and the second term ![]() is the frequency of the next musical note
is the frequency of the next musical note ![]() , Therefore, the fundamental frequency of the note Si is the product between the note Lá by Tom
, Therefore, the fundamental frequency of the note Si is the product between the note Lá by Tom ![]() , as well as the product between the frequency of the note Si by the semitone, results in the frequency of the note Dó
, as well as the product between the frequency of the note Si by the semitone, results in the frequency of the note Dó ![]() and the product of the note frequency Dó by the microtone, results in the frequency of the note Dá
and the product of the note frequency Dó by the microtone, results in the frequency of the note Dá ![]() and so on for any musical note.
and so on for any musical note.
However, it is worth remembering that these frequencies are approximate values, as the Tone ![]() , the Semitone
, the Semitone ![]() and the Microtom
and the Microtom ![]() are irrational or infinite numbers, like the number pi
are irrational or infinite numbers, like the number pi ![]() Furthermore, for a better operational understanding of frequency intervals on a linear scale, one Semitone is considered equal to one-half Tone
Furthermore, for a better operational understanding of frequency intervals on a linear scale, one Semitone is considered equal to one-half Tone ![]() a Microtom is equal to a quarter of Tone
a Microtom is equal to a quarter of Tone![]() and a semitone equal to half a microtone
and a semitone equal to half a microtone ![]() .
.
2.3 REPRESENTATION OF MUSICAL TIMBRE
When the fundamental frequency ![]() is taken from the set of harmonic frequencies, which make up any frequency
is taken from the set of harmonic frequencies, which make up any frequency ![]() the remaining frequencies will form the Timbre
the remaining frequencies will form the Timbre ![]() from Latin Timpanum, which is a secondary characteristic of the resulting frequency
from Latin Timpanum, which is a secondary characteristic of the resulting frequency ![]() , but that allows us to distinguish distinct sounds with the same fundamental frequency.
, but that allows us to distinguish distinct sounds with the same fundamental frequency.
This feature is considered optional in Musical Algebraic Language, leaving it up to the performer to choose the instrument to be used in the emission of musical notes. However, the Timbre can be represented by a natural integer number accented with a tilde![]() appended to the musical note cipher
appended to the musical note cipher ![]() or at the beginning of the spelling
or at the beginning of the spelling ![]() for every melody or come before a parenthesis only for some musical notes
for every melody or come before a parenthesis only for some musical notes ![]() , where
, where ![]() is the melody part indicating the instrument to be used is subtitled (
is the melody part indicating the instrument to be used is subtitled (![]() = instrument).
= instrument).
2.4 REPRESENTATION OF MUSICAL RANGE
The amplitude (a) of a musical note ![]() is measured by its sound intensity in decibels
is measured by its sound intensity in decibels![]() , which can vary from zero to an audible limit bearable by the human being (HELERBROCK, s.d.), being the characteristic that allows us to distinguish a weak sound from a strong sound.
, which can vary from zero to an audible limit bearable by the human being (HELERBROCK, s.d.), being the characteristic that allows us to distinguish a weak sound from a strong sound.
Normally, the sound intensity range between forty to sixty decibels is used ![]() as the normal intensity range for any musical note to be heard by human beings and, below this range
as the normal intensity range for any musical note to be heard by human beings and, below this range ![]() , the cipher of the note is identified with a grave accent
, the cipher of the note is identified with a grave accent ![]() and, above this range
and, above this range ![]() with an acute accent
with an acute accent ![]() . Also, if a specific intensity is needed for any value, just a circumflex accent on that value attached to the note cipher
. Also, if a specific intensity is needed for any value, just a circumflex accent on that value attached to the note cipher ![]() , indicating this intensity multiplied by ten
, indicating this intensity multiplied by ten![]() , may also come before a parenthesis for several musical notes
, may also come before a parenthesis for several musical notes ![]() . An arrow attached to a musical note will indicate that its sound intensity is increasing
. An arrow attached to a musical note will indicate that its sound intensity is increasing ![]() descending
descending ![]() or increasing-decreasing and vice-versa
or increasing-decreasing and vice-versa![]() .
.
2.5 REPRESENTATION OF MUSICAL DURATION TIME
the duration time ![]() of a musical cell
of a musical cell ![]() , note or rest, is the characteristic that allows us to distinguish a sound of short duration from a sound of long duration, being represented by a positive natural integer number
, note or rest, is the characteristic that allows us to distinguish a sound of short duration from a sound of long duration, being represented by a positive natural integer number ![]() , that in a melodic arrangement before the cipher of a musical cell
, that in a melodic arrangement before the cipher of a musical cell ![]() , represents a duration equal to or greater than one second
, represents a duration equal to or greater than one second ![]() . In this context, the most used are those of a second
. In this context, the most used are those of a second ![]() , two seconds
, two seconds ![]() , three seconds
, three seconds ![]() , four seconds
, four seconds ![]() and few others over that duration
and few others over that duration ![]() .
.
Due to the most used measures in a musical rhythm, in this study, the standard period is limited to four seconds![]() and, when that same integer comes in a melodic arrangement after the cipher of a musical cell
and, when that same integer comes in a melodic arrangement after the cipher of a musical cell ![]() it will represent a duration equal to or less than one second
it will represent a duration equal to or less than one second ![]() constituting a fractional number to Music.
constituting a fractional number to Music.
Implying “n” as a denominator number of a fraction, whose numerator is the unit ![]() , the most used durations being half a second
, the most used durations being half a second ![]() , a third of a second
, a third of a second ![]() , a quarter of a second
, a quarter of a second ![]() , one sixth of a second
, one sixth of a second ![]() , an eighth of a second
, an eighth of a second ![]() and no more than a ninth of a second long
and no more than a ninth of a second long ![]() , because the sound ceases to be auditory for the human being when it is equal to or less than a tenth of a second
, because the sound ceases to be auditory for the human being when it is equal to or less than a tenth of a second ![]() . Therefore, both the duration time in seconds and in fractions of a second can be represented in the same musical cell. For example,
. Therefore, both the duration time in seconds and in fractions of a second can be represented in the same musical cell. For example, ![]() is the note Ré three seconds long,
is the note Ré three seconds long, ![]() is the note Ré one-third of a second long and
is the note Ré one-third of a second long and ![]() is the note Ré one second, another half and another quarter of a second long.
is the note Ré one second, another half and another quarter of a second long.
When a duration time ![]() come with an acute accent
come with an acute accent ![]() or serious
or serious ![]() , means it is instantaneous or equal to one ninth of a second
, means it is instantaneous or equal to one ninth of a second ![]() , with the rest of the actual duration in musical pause. In this case, the acute accent indicates an amplitude with strong intensity and the bass accent indicates an amplitude with weak intensity. For example,
, with the rest of the actual duration in musical pause. In this case, the acute accent indicates an amplitude with strong intensity and the bass accent indicates an amplitude with weak intensity. For example, ![]() it is the note Dó with strong sound intensity in the instantaneous duration
it is the note Dó with strong sound intensity in the instantaneous duration ![]() and pause for the remainder of the half-second duration.
and pause for the remainder of the half-second duration.
When a duration is followed by a colon ![]() it means a brief interruption in the musical composition, allowing the musicians to interact with the audience, restarting at any time, normally in the same measure of the interruption.
it means a brief interruption in the musical composition, allowing the musicians to interact with the audience, restarting at any time, normally in the same measure of the interruption.
When a duration is accompanied by an ellipsis ![]() means that after its end, it extends in musical pause for another certain time, being able to end in the same measure or in another measure different. For example,
means that after its end, it extends in musical pause for another certain time, being able to end in the same measure or in another measure different. For example, ![]() it is the note Sol with a duration of one second, and can end in the same measure or in any other measure.
it is the note Sol with a duration of one second, and can end in the same measure or in any other measure.
When a musical note starts in one measure and ends in another without losing its sound continuity, its cipher in the next measure is identified with an apostrophe. For example, the note Dó with three seconds ![]() , being two seconds
, being two seconds ![]() in a measure and a second
in a measure and a second ![]() in the next bar
in the next bar ![]() .
.
3. OPERATIONS BETWEEN MUSICAL CELLS
The operations between musical cells form groupings of notes and musical rests, called musical monomials and when a duration time ![]() come between two musical cells
come between two musical cells ![]() , it will always belong to the first cell
, it will always belong to the first cell ![]() and the second has a later duration. In this case, it’s a second implied
and the second has a later duration. In this case, it’s a second implied ![]() and only a comma before that duration
and only a comma before that duration![]() will make you belong to the second cell
will make you belong to the second cell ![]() making the first one last for an implied second
making the first one last for an implied second![]() . For example,
. For example, ![]() it is the note Dó with half a second and the note Mi one second long and
it is the note Dó with half a second and the note Mi one second long and ![]() it is the note Dó with a second and the note Mi two seconds long.
it is the note Dó with a second and the note Mi two seconds long.
3.1 OPERATION “M” BETWEEN MUSICAL CELLS
When any two or more musical cells are in operation M, they form a melodic algebraic monomial m, the result of which is a melody between them ![]() , where the final duration time of the first note
, where the final duration time of the first note ![]() is equal to the initial duration time of the second note
is equal to the initial duration time of the second note ![]() , as well as the final duration time of the second note
, as well as the final duration time of the second note ![]() is equal to the initial duration time of the third note
is equal to the initial duration time of the third note ![]() . For example, musical notes Dó, Mi, Sol, Mi, Dó, all one second long
. For example, musical notes Dó, Mi, Sol, Mi, Dó, all one second long ![]() in Operation M form a melodic monomial of domisolmidó melody
in Operation M form a melodic monomial of domisolmidó melody ![]() and, any change of position of one of these notes, in this operation, will form another result with another melody
and, any change of position of one of these notes, in this operation, will form another result with another melody ![]() .
.
3.2 OPERATION “H” BETWEEN MUSICAL CELLS
When two or more rhythmic musical notes or notes of equal duration ![]() stay in operation
stay in operation ![]() , they form a harmonic algebraic monomial h, the result of which is a harmony between them, where their initial duration times are all equal
, they form a harmonic algebraic monomial h, the result of which is a harmony between them, where their initial duration times are all equal ![]() , as well as their final duration times
, as well as their final duration times ![]() .
.
Equal musical notes, in this operation, result in a unison monomial or a single musical note![]() and, when these notes are arrhythmic or with different durations, they will form various harmonies depending on the operation M. For example, the operation H in between
and, when these notes are arrhythmic or with different durations, they will form various harmonies depending on the operation M. For example, the operation H in between![]() and between
and between ![]() . In this case, the note Sol with shorter duration forms the first harmony domisol
. In this case, the note Sol with shorter duration forms the first harmony domisol ![]() , leaving the Mi note with a shorter duration
, leaving the Mi note with a shorter duration ![]() forming the harmony domi
forming the harmony domi ![]() in melody with the domisol harmony, leaving the note Dó one second long
in melody with the domisol harmony, leaving the note Dó one second long ![]() in melody with harmony domi.
in melody with harmony domi.
3.3 OPERATION “M” BETWEEN MUSICAL MONOMIS
When two or more musical monomials are in operation ![]() , they will form a melodic polynomial
, they will form a melodic polynomial ![]() , where their monomials are separated by white spaces, implying the operators
, where their monomials are separated by white spaces, implying the operators ![]() between them, being able to be formed: only by melodic monomials in a single melody
between them, being able to be formed: only by melodic monomials in a single melody ![]() , only by harmonic monomials in a melody of harmonies
, only by harmonic monomials in a melody of harmonies ![]() and composed of melodic and harmonic monomials
and composed of melodic and harmonic monomials ![]() .
.
These monomials must be rhythmic or have equal periods ![]() , being determined by the sum of the duration times of their musical cells
, being determined by the sum of the duration times of their musical cells ![]() , property called in this study the Rhythm Principle, which allows any system to remain in equilibrium during its existence and, even if an eventual arrhythmia occurs in its structure or if one of these periods is different from the others, the following period will remain the same as the previous one. equilibrium period
, property called in this study the Rhythm Principle, which allows any system to remain in equilibrium during its existence and, even if an eventual arrhythmia occurs in its structure or if one of these periods is different from the others, the following period will remain the same as the previous one. equilibrium period ![]() to correct the occasional imbalance. However, a sequence of arrhythmias in the rhythmic structure can cause the rhythm of this polynomial to collapse.
to correct the occasional imbalance. However, a sequence of arrhythmias in the rhythmic structure can cause the rhythm of this polynomial to collapse.
When the monomials of this polynomial are arrhythmic, they must be modulated in a rhythm in a binary rhythmic structure ![]() , ternary
, ternary ![]() , quaternary
, quaternary ![]() or any other
or any other ![]() , adjusting the arrhythmic periods in rhythmics and only the first and the last monomial of a rhythm can be incomplete of musical notes, respectively, subtending musical pause before and after these notes completing the periods of these monomials, as shown in the examples below.
, adjusting the arrhythmic periods in rhythmics and only the first and the last monomial of a rhythm can be incomplete of musical notes, respectively, subtending musical pause before and after these notes completing the periods of these monomials, as shown in the examples below.
a) Operation ![]() with the rhythmic monomials
with the rhythmic monomials![]()
![]()
![]() quaternary melodic polynomial
quaternary melodic polynomial
b) Operation ![]() with the arrhythmic monomials
with the arrhythmic monomials
3.4 OPERATION “H” BETWEEN MUSICAL MONOMIS
When two or more musical monomials are in operation ![]() , they form a harmonic polynomial
, they form a harmonic polynomial ![]() . Thus, the monomials remain in the same operational form, separated by their operators
. Thus, the monomials remain in the same operational form, separated by their operators ![]() or they can be in the form of a column array
or they can be in the form of a column array 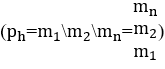 , can be formed only by melodic monomials in harmony
, can be formed only by melodic monomials in harmony ![]() , only by harmonic monomials
, only by harmonic monomials ![]() and composed of melodic and harmonic monomials
and composed of melodic and harmonic monomials ![]() . Below is an example of the Operation
. Below is an example of the Operation ![]() between three rhythmic musical monomials.
between three rhythmic musical monomials.
Below is an example of the Operation ![]() between three rhythmic musical monomials.
between three rhythmic musical monomials.
The harmonic monomial ![]() can be replaced by its chord form of Dó larger
can be replaced by its chord form of Dó larger![]() (ALMADA, 2012). In this way, its duration is implied and is equal to the duration of the melodic monomials of this operation
(ALMADA, 2012). In this way, its duration is implied and is equal to the duration of the melodic monomials of this operation ![]() or it can stick to its specific duration if it is different from the duration of the melodic monomial, which determine the period of the bar. For example, if
or it can stick to its specific duration if it is different from the duration of the melodic monomial, which determine the period of the bar. For example, if ![]() .
.
3.5 OPERATION “M” BETWEEN MUSICAL POLYNOMIS
When two or more rhythmic musical polynomials are in operation M, they can form various types of new monorhythmic musical polynomials. When all the polynomials present the same rhythm, otherwise, they form polyrhythmic polynomials, being the most used in Music, those formed only by harmonic polynomials that are rhythmic compounds ![]() , as example below.
, as example below.
Given the compound polynomials form: ![]()
3.6 OPERATION “H” BETWEEN MUSICAL POLYNOMIS
When two or more rhythmic musical polynomials are in operation ![]() ,they can form several types of new musical polynomials, the most used in Music being those formed between melodic polynomials only with rhythmic melodic monomials, with melodic polynomials only with rhythmic harmonic monomials
,they can form several types of new musical polynomials, the most used in Music being those formed between melodic polynomials only with rhythmic melodic monomials, with melodic polynomials only with rhythmic harmonic monomials ![]() , in this case, the monomials of the operating polynomials
, in this case, the monomials of the operating polynomials ![]() must be rhythmic with each other, as shown in the example below.
must be rhythmic with each other, as shown in the example below.
Given the melodic polynomials form ![]()
4. GRAPHICS OF A MUSICAL POLYNOM
Musical monomials can be plotted on a graph on the axes of Cartesian coordinates. To do this, the frequencies or figures of your musical notes must be allocated on the ordinate axis or on its vertical line, and on the abscissa axis or on its horizontal line, the duration time of these notes. Then, connecting these points with line segments for a melodic monomial, the Melodic Line graph is formed.
However, for a harmonic monomial, where their durations are simultaneous, simply plot the duration of its tonic musical note or the main note of the musical accompaniment chord on the melodic monomial line graph, just above the figure for a harmony of the major and a little lower for a harmony of the minor mode, forming the Harmonic Line graph, according to the examples listed below.
Graphic 1. Musical scale in C in binary rhythm
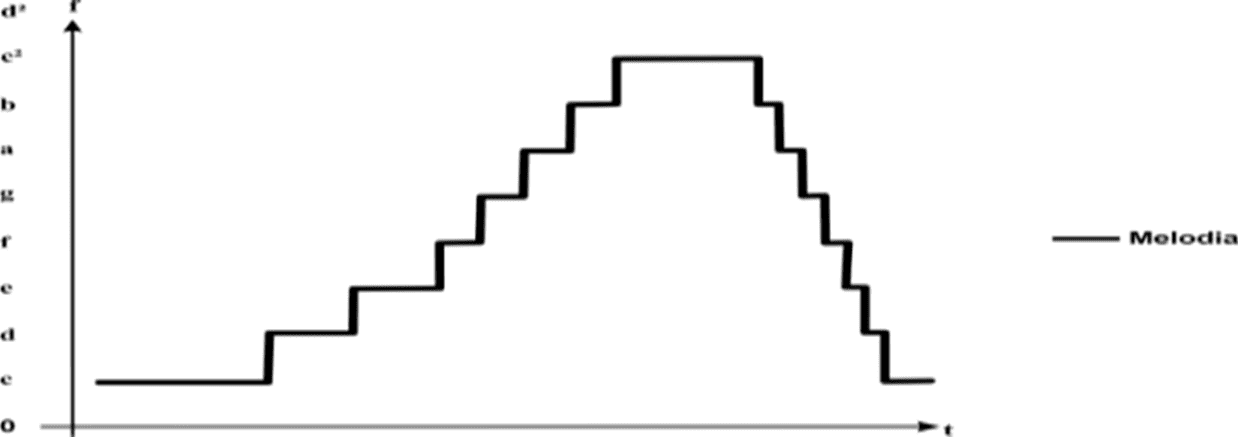
Graphic 2. Simple melody in quaternary rhythm.
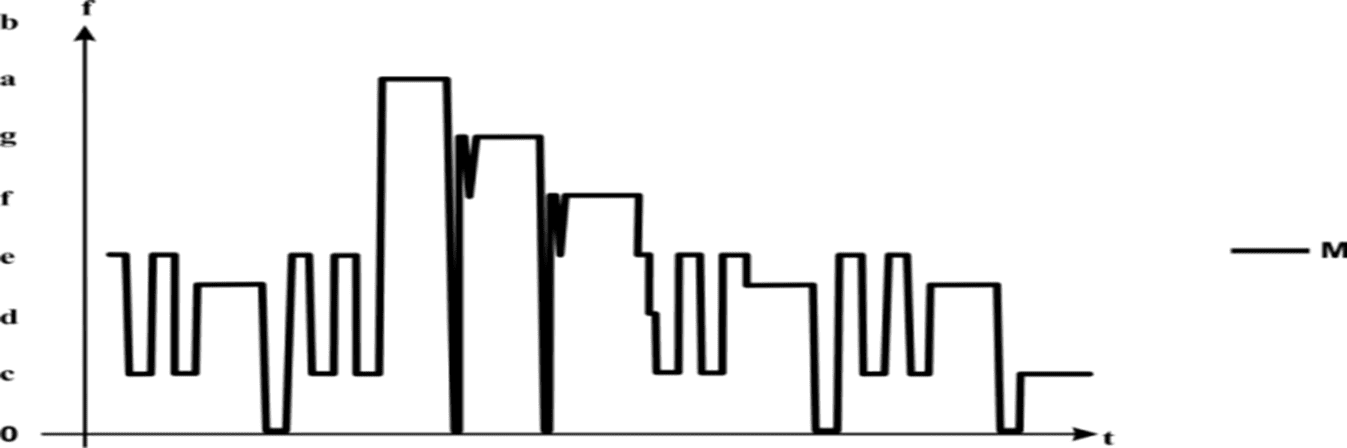
Graphic 3. Melody with a harmony.
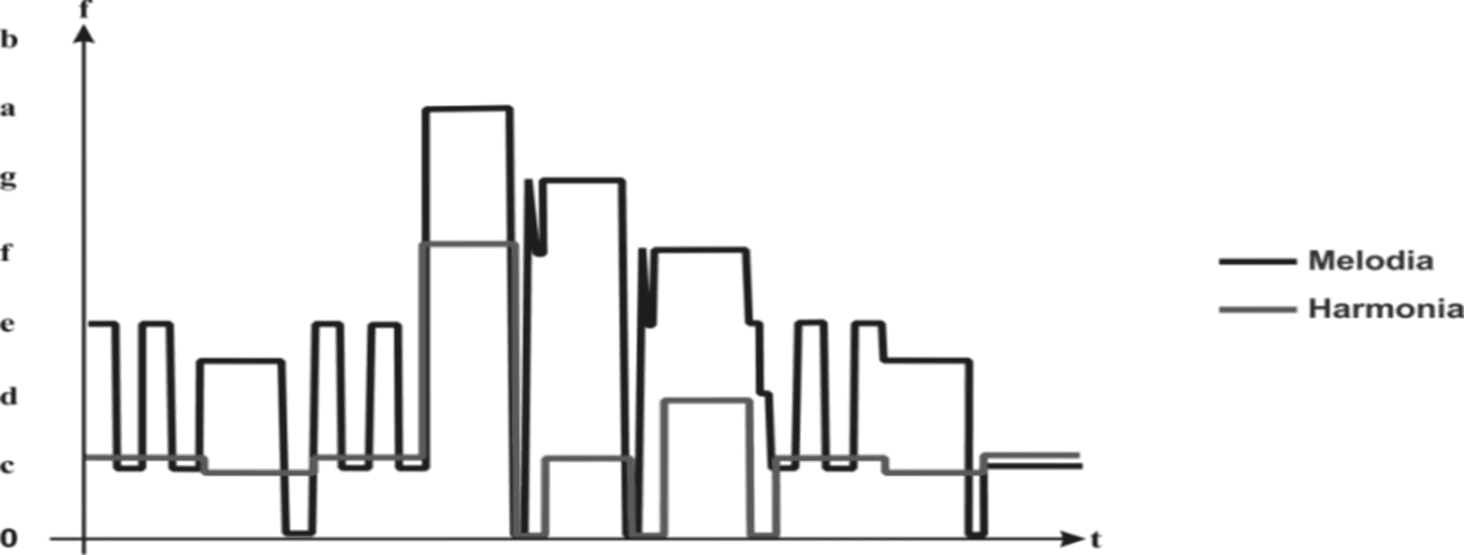
Caption: e=melody; c=harmony
Source: author.
5. HARMONY OF MUSICAL ACCOMPANIMENT
Given any melody ![]() , you can determine your musical accompaniment harmony by applying the Harmonic Module
, you can determine your musical accompaniment harmony by applying the Harmonic Module ![]() developed in this study. In each measure of this melody, which transforms melodic musical notes into harmonics, through the operation
developed in this study. In each measure of this melody, which transforms melodic musical notes into harmonics, through the operation ![]() between them
between them ![]() , whose resulting harmony is adjusted to an existing major or minor mode accompaniment chord harmony (ALMADA, 2012), as example of the melody below.
, whose resulting harmony is adjusted to an existing major or minor mode accompaniment chord harmony (ALMADA, 2012), as example of the melody below.
Adjustment of ![]() to the chord of Dó major:
to the chord of Dó major: ![]()
Adjustment of ![]() to the Dó minor chord as a function of
to the Dó minor chord as a function of ![]()
Adjustment of ![]() to the Fá major chord:
to the Fá major chord: ![]()
Adjustment of ![]() to the chord of Dó major:
to the chord of Dó major: ![]()
Adjustment of ![]() to the chord of Ré minor:
to the chord of Ré minor: ![]()
The accompanying harmony ![]() it’s a simple harmony. In the example above, the harmonies found in the harmonic modules were adjusted to the most suitable existing major and minor chords, taking into account the influence of the tonic note of the chord of melody Dó major
it’s a simple harmony. In the example above, the harmonies found in the harmonic modules were adjusted to the most suitable existing major and minor chords, taking into account the influence of the tonic note of the chord of melody Dó major ![]() . As demonstrated, in some bars, musical notes of short duration were left without musical chords, without harming the harmony
. As demonstrated, in some bars, musical notes of short duration were left without musical chords, without harming the harmony ![]() of the melody
of the melody ![]() .
.
5.1 MUSICAL ACCOMPANIMENT MELODY
These musical accompaniment harmonies can be turned into an accompaniment melody. To do this, simply apply the Melodic Module to these harmonies ![]() , which transforms a harmony into several melodies through Operation
, which transforms a harmony into several melodies through Operation ![]() between your harmonic notes
between your harmonic notes![]() , that for a triad or three musical notes in harmony
, that for a triad or three musical notes in harmony ![]() , will result in six distinct melodies
, will result in six distinct melodies ![]() .
.
Due to the permutation between these three musical notes to form the note arrangements in melodies ![]() and for a tetrad or four musical notes
and for a tetrad or four musical notes ![]() , will result in twenty-four distinct melodies, due to the permutation of four being equal to 24 melodic arrangements
, will result in twenty-four distinct melodies, due to the permutation of four being equal to 24 melodic arrangements ![]() . From there, just choose one of these melodies for the musical accompaniment of the melodic monomial of this harmony, subtitling it separately. In this case, the duration time of each note is determined by the inverse of the number of musical notes per second
. From there, just choose one of these melodies for the musical accompaniment of the melodic monomial of this harmony, subtitling it separately. In this case, the duration time of each note is determined by the inverse of the number of musical notes per second ![]() , than for a triad or three musical notes (n=3), is a third of a second long
, than for a triad or three musical notes (n=3), is a third of a second long ![]() , as example below.
, as example below.
Graphic 4. Melody &4 with an accompanying melody.
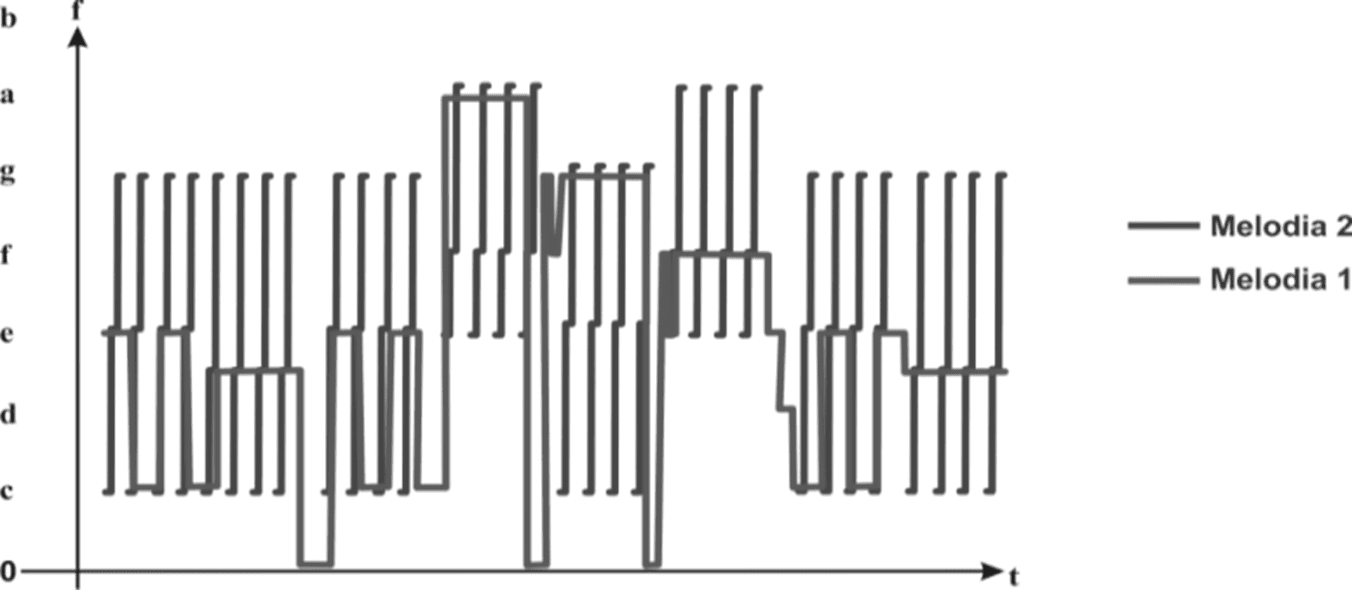
6. MEASURES OF MUSICAL MONOMIS
The musical monomials of a melody or a harmony in general will provide two types of musical measures important to Music, one called Musical Period, which measures the duration of a melodic monomial ![]() , harmonic
, harmonic![]() and composed of melodic with harmonic
and composed of melodic with harmonic ![]() , scaled in seconds
, scaled in seconds ![]() ; and another called Musical Texture
; and another called Musical Texture![]() , which measures the appearance of musical notes in the structure of a melodic monomial
, which measures the appearance of musical notes in the structure of a melodic monomial ![]() , harmonic
, harmonic ![]() or compound
or compound![]() , scaled in musical notes
, scaled in musical notes ![]() . The ratio between Melodic Texture and Melodic Period
. The ratio between Melodic Texture and Melodic Period ![]() determines the Melodic Dynamics of musical notes in the structure of a melodic monomial.
determines the Melodic Dynamics of musical notes in the structure of a melodic monomial.
6.1 PERIOD AND TEXTURE OF A MELODIC MONOMIUM
A melodic monomial ![]() presents its musical notes continuously distributed in space-time, forming a Melodic Period
presents its musical notes continuously distributed in space-time, forming a Melodic Period ![]() , determined by the sum of the duration times of its musical notes
, determined by the sum of the duration times of its musical notes ![]() , scaled in seconds
, scaled in seconds ![]() . The Melodic Texture
. The Melodic Texture ![]() , is determined by the number
, is determined by the number ![]() of musical notes
of musical notes ![]() in your melodic period.
in your melodic period.
The Ratio between Melodic Texture and Melodic Period ![]() determines the melodic dynamics of musical notes per second
determines the melodic dynamics of musical notes per second ![]() in the structure of this monomial, as shown below.
in the structure of this monomial, as shown below.
Melodic texture: ![]() four musical notes
four musical notes
Melodic texture: ![]() sixteen musical notes
sixteen musical notes
Melodic dynamics: ![]() very fast melody
very fast melody
6.2 PERIOD AND TEXTURE OF A HARMONIC MONOMIUM
A harmonic monomial ![]() presents its musical notes interconnected at the same time and they are usually rhythmic or of the same duration. Therefore, its Harmonic Period
presents its musical notes interconnected at the same time and they are usually rhythmic or of the same duration. Therefore, its Harmonic Period ![]() is equal to the duration time of any musical note in its structure
is equal to the duration time of any musical note in its structure ![]() , scaled in seconds
, scaled in seconds ![]() .
.
However, it can happen that a harmonic monomial is arrhythmic or presents its musical notes with different durations. In this case, several different harmonies will occur, with different harmonic periods and the melody between them results in the total harmonic period of this monomial ![]() . In addition, the Harmonic Texture
. In addition, the Harmonic Texture ![]() , is determined by the number of musical notes
, is determined by the number of musical notes ![]() in harmony in the harmonic period, as shown below.
in harmony in the harmonic period, as shown below.
2) Arrhythmic harmonic monomial ![]()
6.3 PERIOD AND TEXTURE OF A MELODIC POLYNOMIUM
A rhythmic melodic polynomial is one where its monomials have equal periods. In this way, the product of the number ![]() of bars for its period
of bars for its period ![]() , determines the duration time of this polynomial
, determines the duration time of this polynomial![]() , scaled in seconds or minutes.
, scaled in seconds or minutes.
Even if this polynomial is composed with melodies and harmonies, the calculation of its duration would be the same, while its total melodic texture ![]() remains the number of musical notes in that Duration Time.
remains the number of musical notes in that Duration Time.
The ratio of this Total Melodic Texture to the Total Duration Time forms the Melodic Dynamics ![]() of musical notes in this polynomial, being scaled in musical notes per second
of musical notes in this polynomial, being scaled in musical notes per second![]() .
.
When the melodic polynomial is composed of a harmony, its total Harmonic Texture ![]() is determined by the arithmetic mean of the harmonic textures of all its harmonic monomials of its bars
is determined by the arithmetic mean of the harmonic textures of all its harmonic monomials of its bars ![]() , as shown below.
, as shown below.
Melodic dynamics: ![]() (Melody with slow dynamics)
(Melody with slow dynamics)
All harmonic textures are equal to: ![]()
Total harmonic texture: ![]() (Simple texture)
(Simple texture)
7. PLAGIARISM BETWEEN MELODIC POLYNOMIS
Normally, plagiarisms are equalities that occur between melodic monomials and melodic polynomials ![]() and, how these monomials are formed by musical notes
and, how these monomials are formed by musical notes ![]() continuously distributed that plotted on a graph in the Cartesian coordinate system, show their melodic lines, which can be compared with each other, showing their similarities or differences, those who present their melodic lines are considered plagiarism, that is, that coincide on the graph, placing them one on top of the other, or even transporting the musical notes of the monomials considered plagiarism to the tonality of the notes of the original monomials. Below is an example of plagiarism monomials.
continuously distributed that plotted on a graph in the Cartesian coordinate system, show their melodic lines, which can be compared with each other, showing their similarities or differences, those who present their melodic lines are considered plagiarism, that is, that coincide on the graph, placing them one on top of the other, or even transporting the musical notes of the monomials considered plagiarism to the tonality of the notes of the original monomials. Below is an example of plagiarism monomials.
Equal melodic monomials: ![]() and
and ![]() .
.
Graphic 5. Melodic monomials ![]() .
.
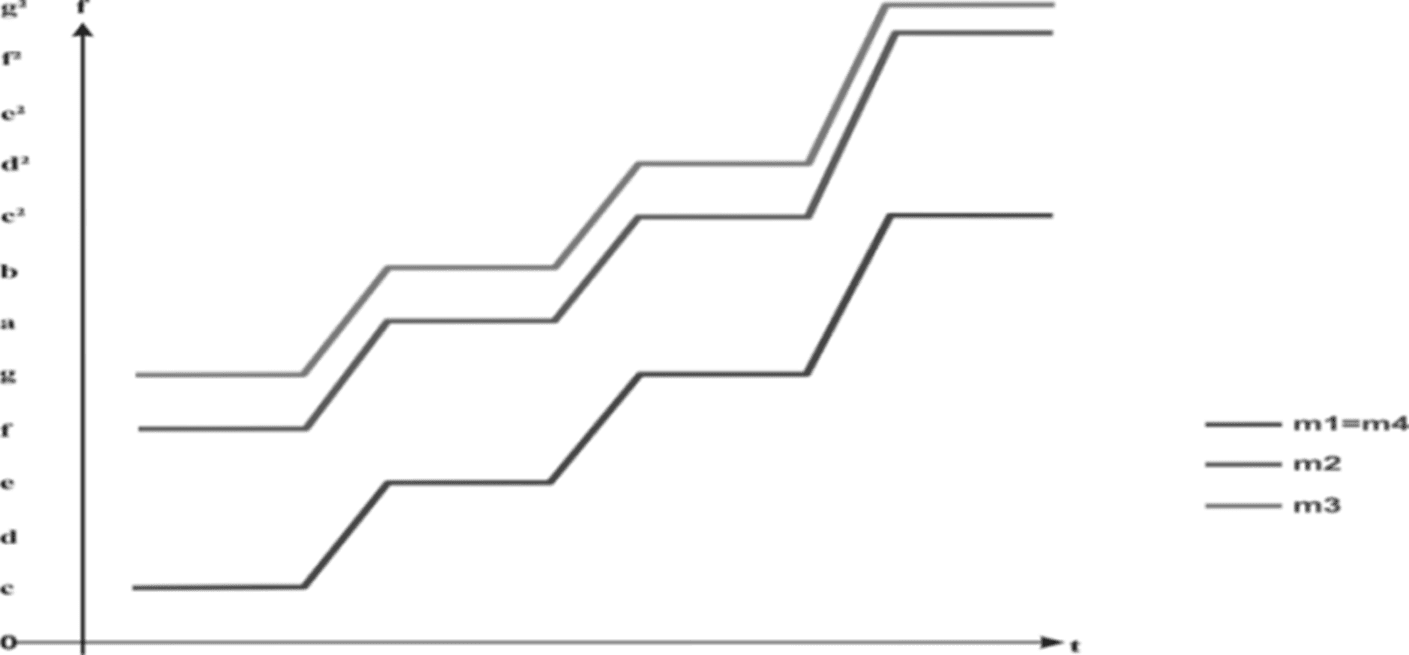
Source: author.
7.1 PLAGIARISM PERCENTAGE
When a continuous part of a melodic polynomial is plagiarism of another, that is, it has a percentage of plagiarism or equality in relation to the original polynomial, the identified similarities can be calculated by the formula ![]() , where
, where ![]() is the number of melodic monomials or bars with plagiarism, and
is the number of melodic monomials or bars with plagiarism, and ![]() is the total number of monomials or measures of the original polynomial, without the melodic repetitions. For example, given a melody with fifty bars and, among them, ten consecutive bars were plagiarized in another tune, so:
is the total number of monomials or measures of the original polynomial, without the melodic repetitions. For example, given a melody with fifty bars and, among them, ten consecutive bars were plagiarized in another tune, so:
8. STRUCTURE OF A RHYTHM
Rhythm is a phenomenon that occurs in the duration of any system, being divided into equal parts to keep itself in balance. This is how it happens in the rhythm of a melody, where its duration ![]() is divided into parts of equal periods, called measures, which are continuously linked as a function of the Operation
is divided into parts of equal periods, called measures, which are continuously linked as a function of the Operation ![]()
![]() , as well, each measure is also divided into equal parts of smaller periods, called units of time
, as well, each measure is also divided into equal parts of smaller periods, called units of time ![]() , which are also continuously interconnected as a function of the Operation
, which are also continuously interconnected as a function of the Operation ![]()
![]() .
.
It is known that the duration of any period ![]() , has an initial duration
, has an initial duration ![]() and final
and final ![]() , soon,
, soon, ![]() . Furthermore, this initial duration
. Furthermore, this initial duration ![]() consists of a beat
consists of a beat ![]() audible or non-audible, caused by an impulse
audible or non-audible, caused by an impulse ![]() , given by a force
, given by a force ![]() for an instantaneous duration, remaining silent for the remainder of its duration
for an instantaneous duration, remaining silent for the remainder of its duration ![]() , therefore, the period of any measure is formed by its initial beat
, therefore, the period of any measure is formed by its initial beat ![]() in tune with your time of silence
in tune with your time of silence ![]() .
.
In a single bar, in addition to the beat of your period with your time of silence ![]() , still exists, in its interior, due to the Operation
, still exists, in its interior, due to the Operation ![]() , the beats of the units of time, with their times of silence
, the beats of the units of time, with their times of silence ![]() , the result of which makes the first beat twice as strong as the others
, the result of which makes the first beat twice as strong as the others ![]() , and the sound effect is called the Rhythmic Cadence of the Measure
, and the sound effect is called the Rhythmic Cadence of the Measure ![]() , that has a beat speed called the Rhythmic Tempo of the Measure, being inversely proportional to the period of this unit of time
, that has a beat speed called the Rhythmic Tempo of the Measure, being inversely proportional to the period of this unit of time ![]() , scaled in beats per second
, scaled in beats per second ![]() or beats per minute
or beats per minute ![]() .
.
In this context, the shorter the duration of this unit of time, the greater the rhythmic tempo of these beats, which provides two rhythmic movements in a physical body in its area of operation, one of which is called Rhythmic Regency ![]() , where a body without leaving its resting position, follows the movement of the Rhythmic Tempo of these beats, and another called Rhythmic Dance
, where a body without leaving its resting position, follows the movement of the Rhythmic Tempo of these beats, and another called Rhythmic Dance ![]() , where a body moves from its resting position to other distinct positions, depending on the rhythmic tempo of these beats.
, where a body moves from its resting position to other distinct positions, depending on the rhythmic tempo of these beats.
8.1 LAW OF TONIC ACCENTUATION OF BARRES
The property of the first beat of the time unit of a measure being stronger than the other beats in the same measure ![]() originated the Law of Tonic Accentuation of the Bars that marks, in an incisive way, any musical note that occupies that first position in the bars of a rhythm. Also accentuating any syllable of any word that occupies that position, modifying or not its orthographic tonic accentuation, whatever the spoken language of the world. In the case of a rest placed in this position and a musical note in the next position, a musical effect called Contratempo occurs.
originated the Law of Tonic Accentuation of the Bars that marks, in an incisive way, any musical note that occupies that first position in the bars of a rhythm. Also accentuating any syllable of any word that occupies that position, modifying or not its orthographic tonic accentuation, whatever the spoken language of the world. In the case of a rest placed in this position and a musical note in the next position, a musical effect called Contratempo occurs.
8.2 STRUCTURE OF A COMPOUND COMPASS
A measure is called a compound when more than one distinct rhythmic cadence occurs in its period ![]() , formed by other distinct time units
, formed by other distinct time units ![]() . In this case, the Compound bar can be either Melodic or Harmonic.
. In this case, the Compound bar can be either Melodic or Harmonic.
In the Melodic Compound Measure, the rhythmic cadences of the time units depend on the Operation ![]()
![]() , resulting in a Melodic Rhythmic Cadence
, resulting in a Melodic Rhythmic Cadence ![]() . Between them originates a melodic rhythmic tempo
. Between them originates a melodic rhythmic tempo ![]() , a melodic rhythmic regency
, a melodic rhythmic regency ![]() and a melodic rhythmic dance
and a melodic rhythmic dance ![]() .
.
In the Harmonic Compound Measure, the rhythmic cadences of the different time units and are depending on the Operation ![]()
![]() , resulting in a Harmonic Rhythmic Cadence between them
, resulting in a Harmonic Rhythmic Cadence between them ![]() .
.
On condition that ![]() , the resulting Rhythmic Cadence is determined by the unit of time with the shortest duration
, the resulting Rhythmic Cadence is determined by the unit of time with the shortest duration ![]() , in this case, the resulting harmonic rhythmic Tempo is given by the period of the smallest unit of time
, in this case, the resulting harmonic rhythmic Tempo is given by the period of the smallest unit of time ![]() , with its rhythmic harmonic conducting
, with its rhythmic harmonic conducting ![]() normally a function of the rhythmic tempo of the largest unit of time
normally a function of the rhythmic tempo of the largest unit of time ![]() and its rhythmic harmonic dance
and its rhythmic harmonic dance ![]() depending on the major and minor rhythmic tempo
depending on the major and minor rhythmic tempo ![]() .
.
8.3 HARMONIC RHYTHM
The Rhythmic Cadence of a bar is usually melodic ![]() , due to its time units being a function of the Operation
, due to its time units being a function of the Operation ![]()
![]() . However, this cadence can be harmonic
. However, this cadence can be harmonic ![]() with the periods of its time units as a function of the Transaction
with the periods of its time units as a function of the Transaction ![]()
![]() , resulting in a single unit of time, with its melodic rhythmic cadence
, resulting in a single unit of time, with its melodic rhythmic cadence ![]() . For example, when a group of people are silent for a certain period of time or when several clocks are in sync.
. For example, when a group of people are silent for a certain period of time or when several clocks are in sync.
9. FINAL CONSIDERATIONS
This article aimed to develop an algebraic language to mathematically structure Music, using only letters, numbers and symbols to write the sounds of musical notes in an alphanumeric way, representing its main sound characteristics, such as: frequency ![]() , amplitude
, amplitude ![]() and duration time
and duration time ![]() , in a single expression
, in a single expression ![]() to identify a sound wave.
to identify a sound wave.
In the end, it appears that the Mathematics of Music was only possible with the development of Operation ![]() and its inverse Operation
and its inverse Operation ![]() , providing the groupings of sound waves of musical notes, respectively in the formations of harmonies of instrumental accompaniments and melodies that appear at all times in the inspiration of a composer, making the study of music simpler, with a sound language more understandable and adapted to current technology of the scientific studies of the Science of Music. Finally, with the demonstration of the Mathematical Structure of Music, it can be said that a musical composition is a mathematical structure of sound.
, providing the groupings of sound waves of musical notes, respectively in the formations of harmonies of instrumental accompaniments and melodies that appear at all times in the inspiration of a composer, making the study of music simpler, with a sound language more understandable and adapted to current technology of the scientific studies of the Science of Music. Finally, with the demonstration of the Mathematical Structure of Music, it can be said that a musical composition is a mathematical structure of sound.
REFERENCE
ALMADA, Carlos. Harmonia Funcional. Editora Unicamp, 2ª Edição, 2012.
GUEST, Ian. Harmonia – Método Prático. Editora Luminar. Vol. 1, p. 33 a 41, 2020.
HELERBROCK, Rafael. Intensidade do som. Mundo da educação, s.d. Disponível em: https://mundoeducacao.uol.com.br/fisica/velocidade-intensidade-som.htm. Acesso em: 22 de julho de 2022.
SILVA, Luiz Paulo Moreira. Progressão geométrica. Brasil Escola, s.d. Disponível em: https://brasilescola.uol.com.br/matematica/progressao-geometrica.htm. Acesso em? 22 de julho de 2022.
VIANA, Arnóbio Araújo. A operação harmonização (H) e sua inversa operação melodiação (M). Revista Científica Multidisciplinar Núcleo do Conhecimento. Ano. 07, Ed. 03, Vol. 03, pp. 144-171. Março de 2022. ISSN: 2448-0959, Link de acesso: https://www.nucleodoconhecimento.com.br/matematica/operacao-harmonizacao, DOI: 10.32749/nucleodoconhecimento.com.br/matematica/operacao-melodiacao. Acesso em: 22 de julho de 2022.
[1] Graduated in Electrical Engineering, op. Electronics from the Federal University of Pará-UFPA. ORCID: 0000-0001-7010-9114.
Sent: July, 2022.
Approved: August, 2022.