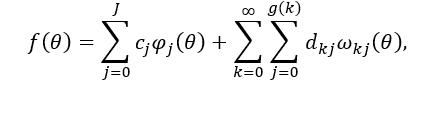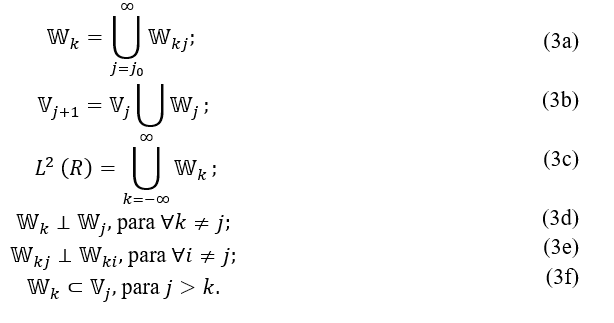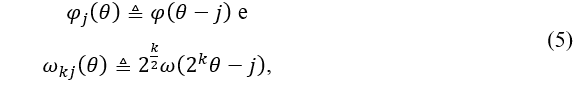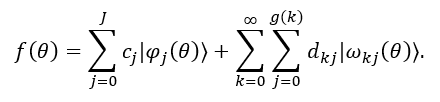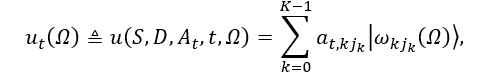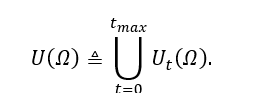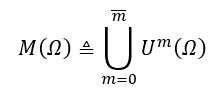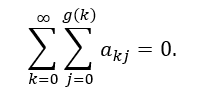A discrete universe as a proposal for the unification of physics

ORIGINAL ARTICLE

ARAÚJO, Leonardo Oliveira de ^[1]

ARAÚJO, Leonardo Oliveira de. A discrete universe as a proposal for the unification of physics. Revista Científica Multidisciplinar Núcleo do Conhecimento. Year. 07, Ed. 02, Vol. 02, p. 122-138. February 2022. ISSN: 2448-0959, Access link: https://www.nucleodoconhecimento.com.br/fisica-en/unification-of-physics, DOI: 10.32749/nucleodoconhecimento.com.br/fisica-en/unification-of-physics

ABSTRACT

This article fits in the context of the researches of general formulations of Physics that propose satisfactory answers for the unification of the theories of Quantum Mechanics and of General Relativity, contributing additionally with the studies that approach a discrete structure of space-time and a quantum theory to gravity. The objective is to present a primary structure in which matter and space-time emerge as possible arrangements, compatible with the observable universe and with the two great theories mentioned. The methodology is based on the Discrete Wavelet Transform, a widespread mathematical tool in the signal processing area, used to model the primary structure from which space-time and particles derive. Unification efforts that compose String Theories and Loop Quantum Gravity are used in this proposal. The results make it possible to meet the stated objective and, additionally, to model dark energy and dark matter. In addition, an experimental observation is proposed to validate this proposal.

Keywords: Unification, Quantum Physics, Relativity, Quantum Mechanics, Wavelet Transform.

1. INTRODUCTION

The Special and General Theories of Relativity (EINSTEIN, 1995; PIATTELLA, 2020; EINSTEIN, 1922) reformulated the understanding of space and time, presenting them as parts of a single structure: the space-time continuum. Furthermore, in the General Theory of Relativity it is established that gravity is the interpretation of the deformation of space-time.

The conclusion that energy has discrete values brought to light a series of experiments, conceptual formulations and mathematical models that, like Relativity, distance themselves from the non-relativistic classical physics (adhering to common sense) that appeared until the end of the 19th century. . This set of concepts is condensed in Quantum Mechanics (STAMATESCU and SEILER, 2007).

Both Relativity and Quantum Mechanics enjoy enormous success in predicting theoretical results if confronted with experiments. Despite other considerations, these two supports of modern physics have their formulations in the space-time continuum.

The widespread success of the application of Field Theory to Quantum Mechanics made it possible to add efficiency and greater understanding to the domain of phenomena and to the studies of this Mechanics. It is this combination that results in the current standard model of Particle Physics.

However, the attempt to apply, in a similar way, Field Theory to General Relativity proved to be frustrating (KUCHA, 1988). This highlighted the difficulty of modeling gravity as the field of a force mediated by a discrete particle, in this case, the graviton.

As a consequence, Quantum Mechanics presents three of the four forces that exist in nature (electromagnetic, strong, weak and gravity) mediated by particles, that is, they are agents of discrete action, but it does not deal with the gravitational force (BOJOWALD, 2015).

General Relativity explains the fourth force (gravity) as a continuous agent of transmission: space-time. More than that, the deformation of space-time (gravity) is caused by the presence of mass (interrelating stage, space-time, and actors, mass and energy): no mass, no deformations.

However, some studies available in the literature (CHIAO, 2003; GREENE, 2001; SMOLIN, 2002; KAKU, 2000) require the combination of both theories. The problem is that this can generate incongruous results, notably, in particular, infinity is obtained as the value of physical quantities (absurd). Furthermore, from Quantum Mechanics, the Uncertainty Principle leads to the conclusion that, in an absolute vacuum, any fields (including gravitational ones), on a sufficiently small scale, must oscillate (randomly). Indeed, there would be generation and variation of gravitational field without the presence of mass, which clashes with the General Theory of Relativity.

That said, it appears that there is a deep basic structural disagreement regarding causes and effects in the joint use of Quantum Mechanics and Relativity (CHIAO, 2003), a problem that must be solved in order to obtain a unified model that correlates physical effects , observations and theories (GREENE, 2001; SMOLIN, 2002; KAKU, 2000).

Feynman says that the only true test of a scientific theory is experimentation (FEYNMAN, 1965). Taking this as a premise, it is necessary to focus on the success and precision of Relativity and Quantum Mechanics as beacons for any new theory that seeks to unify them.

One way to guarantee the aforementioned alignment is to keep space-time as the transmission element of gravity information, that is, the agent through which the presence of this basic force of nature is perceived is the deformation of space-time. In parallel, the discrete treatment applied to the other force mediators must be maintained, which leads several studies to a discrete formulation of the gravitational mediator (SCHULZ, 2014; BOJOWALD, 2015).

Indeed, a possible solution coherent with such pillars will present a space-time discretization. The scientific literature brings some examples (DOPLICHER et al., 1994) in which space-time starts to be perceived as Quantized Space-Time (ETQ). It is essential that the ETQ presents a characteristic that, in large-scale interpretations, that is, in dimensional segments compatible with those involved in the experiments carried out up to the current stage, is equivalent to a deformation in space-time if this is assumed, even in a approximation, as continuous.

Thus, it can be stated that the physical laws must be represented by a model that is valid in relation to experiments and approximations considered in other theories that present more restricted validity of use (theoretical covariance).

Note that, ultimately, theoretical covariance results in a profound unification, in the sense that it points to the need for the ETQ to be defined by the same basic set of information that defines matter-energy.

It is not the intention of this article to present adjustments or adjustments to the equations that model gravity or other forces. But the objective is to define the physical-mathematical model of a generic point in the universe to enable the determination of such equations. Therefore, in addition to this introduction, this manuscript is structured as follows: a presentation of Principles of the Discrete Wavelet Transform, basis for the proposed model; the presentation of the main contribution of this article, approaching some particular aspects, in particular considerations about dark matter and energy, and observations that can prove or disprove the presented model; and, finally, a brief conclusion.

2. PRINCIPLES OF DISCRETE WAVELET TRANSFORMATION

The Discrete Wavelet Transform (DWT) is used to represent quadratically integrable functions, that is, formula 1, where formula 2 is the space defined by the Formula 3 functions that satisfy the following condition (MALLAT, 2009):

The Discrete Wavelet Transform (DWT) is used to represent quadratically integrable functions, that is,, , on what is the space defined by the functions that satisfy the following condition (MALLAT, 2009):

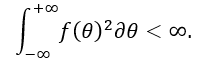
(1)
In this way, a function is represented as:

(2)

on what  make up the basis of the functional subspace are bases of subspaces. The integer and the function , depend on the adopted DWT basis and on the size of the interval of the considered parametric domain D_θ.

These functional spaces have the following characteristics:

The spaces ?₀ and ?_k  are generated by (BURRUS et al., 1998):

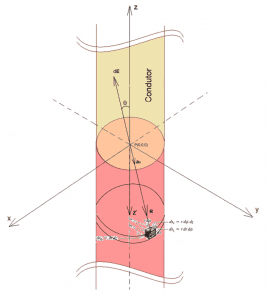
Figure 01: Relationship between spaces ?_j, ?_k e ?_kj.

In equation (3b), and according to Figure 01, it appears that the functional space ?_j  is defined as the orthogonal complement of ?_j about ?_j+1.

The base functions    are obtained as follows:

where j and k ∈  ℕ are, respectively, translation and scaling indexers and and are called parent and parent functions, respectively.(5)

The coefficients   and , used in equation (2), are calculated by the inner product:

(6)

Although it is not a requirement to be a wavelet, in this study, the functions   and of interest are those orthonormal.

From now on, the Dirac notation (DIRAC, 1939), Bra-Ket, will be used to denote the wavelet basis functions. In fact, (2) is rewritten as:

(7)

3. UNIQUENESS PRINCIPLE

In the present study, assumptions (P) and boundary conditions (CC) are used to establish a standard model, to be defined in the subsequent equations, and to apply to it what is verified in the known universe.

Before presenting the definition of the principle of uniqueness, it is necessary to introduce the following premise:

Premise P 01: the universe can be modeled from a single physical quantity and its variations. The aforementioned greatness is called arché, a term used by Pre-Socratic philosophers to designate the original substance (SPINELLI, 2002).

Arché and a generic image of this magnitude are denoted, respectively, as

The principle of uniqueness is defined as:

– Arché is the basic physical quantity of nature, from which the others derive;

– The physical dimensions are discrete and can be represented by orthonormal basis functions;

– The particles have their properties defined by the vector of coefficients A (whose components are associated with the mentioned functions); and

– The causes and effects that relate particles and dimensions define the laws of nature.

In effect, consider a hypothetical discrete universe for which:

–  defines the spatial dimensions, where k enumerates these dimensions;

– , in which formula defines the spatial coordinate associated with dimension k, that is, defines a point in space; and

–  gathers all the information that defines a particle.

In this study, positive and negative polarity designate, respectively, and .

Then, it is possible to represent the k-th spatial dimension (Sk) using a basis function of the DWT   to model this dimension, with k representing it and j the coordinate, the spatial location, in Sk.

Thus, it is possible to initially define as a set formed of arché images, , and candidate to model the observable universe. A subset is defined, , to which it belongs , a generic point in the universe with K spatial dimensions. Such a point can be modeled and defined by:

(8)

on what . The variable  is the same variable j that represents the displacement in the DWT: the sub-index was added due to the inexistence of the sum in j, in equation (8), signaling the possibility of this index varying as a function of k.

In the particular case where  , respectively, expanded spatial dimensions and unitary spatial dimensions, for:

– ; and

– Other cases, .

In the particular case of the observed universe, K*=3.

Then,   is the generalization of the points that describe any element of  covering all extensions of S. Consequently, models a subset that spans any point in the K spatial dimensions of .

As equation (8) represents a generic point in the universe with K spatial dimensions in the subset enumerated by t, the subsets   represent the possible variations that occur in S space and, therefore,   models any “t” moment of . Indeed, using as the variable that denotes the sequence of variations that occur in the spatial dimensions, one can define the universe  how:

(9)

That said, it is necessary to describe how the coefficients of A, which define the particles and are present in any element belonging   

, allow the existence of a vacuum.

Boundary Condition CC 01: the empty space, the vacuum, presents intense variations of fields in reduced scales.

In fact: t is equivalent to the (discrete) dimension in which the change in the K spatial dimensions is verified, that is, it is the unit that is equivalent to Planck’s time; and the vacuum is a consequence of the random variation (non-currents) of the coefficients belonging to A in  defined regions of S along(resulting in Quantum Fluctuation).

Thus, the effective identification of a particle in   is given by the coefficients belonging to vector A coherently constituted in time intervals   and of space (staying the same in trajectories described in k, j and t). From the above, the particle is also represented by:

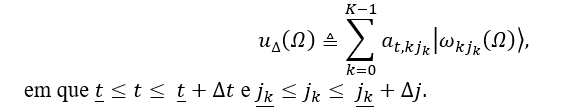
(10)

Given the modeling presented in equation (10), a hypothetical universe can be defined where, at least for some   , this universe contains a subset that differs from any representation of    . Then, from the arché magnitude, it is possible to generalize the representation of the multiverse   as the union of the set of all universes (classical, after the observation of superimposed states) possible to be represented by , i.e:

(11)

Thus, space, time and particles result from interpretations, with particular approaches, of a single base structure, represented by equation (10).

to add characteristics of interest that make it possible to model universes described by Quantum Mechanics and Relativity, such as ours, two models are proposed.

3.1 MODEL 1

This model uses the mapping of parameters from String Theory (POLCHINSKI, 1998; BARBÓN, 2004; ABDALLA, 2005), or from Superstring Theory, in the description presented in equation (8). In that case:

– The amount of spatial dimensions foreseen in the String Theory variant considered, added by any dimension(s) due to the need to express parameters (such as closed strings, loops), is given by K; and

– The coefficients mirror the frequency at which the string vibrates in the dimension A robust advantage of this modeling is that String Theory becomes a background-independent theory (that is, space-time also becomes the object of explanation of the Theory, in this case, the ETQ).

3.2 MODEL 2

Considering equations (8) and (10), the term Spaj is used in this article to designate effects of coefficients of vector A (in whole or in part) related to Sk and that define their effects in it.

Premise P 02: Spaj affects Sk, that is: has its action on .

If has particles as force mediators, the present modeling would result in something similar to Model 1 and would not explain the action of sources of gravity in space-time, pillar of General Relativity (although it may explain gravity as the exchange of graviton). Then, given P 02, in Model 2 it is required the description of how Sk acts as a force mediator, replacing the virtual particles. This explanation is similar to the one presented in General Relativity.

Boundary Condition CC 02: mass is a quantity that is conventionally positive and a source of gravity.

Following the modeling of  , defines itself

as, respectively, the three extended (traditional) spatial dimensions (compatible with what is seen in our physical universe) and eventual additional spatial dimensions necessary to explain properties of the universe.

Then, given P 01, P 02, CC 01, CC 02 and the fact that gravity acts on Sk₀, we have that the mass results from the components of  , here arbitrated as positive polarity. Consequently, in order not to be restrictive to the modeling in progress, it is assumed the existence of    of negative polarity.

How positively polarized has the effect of gravity, one can generalize this identification and, with that, obtain two conclusions:

– Gravity results from the same polarity of   ; and

– Different polarities (consistent with generates antigravity.

This proposed generalization, according to equation (10), has the potential to explain and model Cosmic Inflation (GUTH and STEINHARDT, 1984) and the effects attributed to dark matter (CORBELLI and SALUCCI, 1999; FENG, 2010) and dark energy ( RIESS et al., 1998) from the properties of   : dark matter is modeled by   negatively polarized, while the effects related to dark energy and Cosmic Inflation are modeled by alternating polarity regions of .

In the case of the three extended spatial dimensions, the gravitational and antigravitational actions on Sk are verified as an interpretation of:

– Gradient: the existence of  (identical polarity coefficients) induce gravitational field flux, the very fabric of the ETQ (contraction of these dimensions), for such particles; and

– Divergent: the existence of (inverse polarity coefficients) induce an antigravity field source, the very fabric of the TSQ (space-time stretch), between the particles.

The convergence of the ETQ flux has an intensity equivalent to the (discrete) curvature of space-time presented in General Relativity. In addition, the absence of said polarization results in spatio-temporal distension.

However, these models are completely arbitrary if there is no law or property that defines some limit or rule for . Thus, considering that the generation of a particle also results in the generation of an antiparticle, that the proposal is that A (in this model) defines the properties that characterize them and that it is possible to base the 1st Law of Thermodynamics in this model, it is proposed to premise presented below.

Premise P 03: the sum of the coefficients of the spatial dimensions at an instant t is zero:

(12)

So each coefficient positive has a negative equivalent (or a sum that equals it) and vice versa.

Although for sufficiently large that it is possible to establish a means of meeting P 03 and canceling gradients, given the proportional existence of coefficients   and given the boundary conditions that led to the concepts of dark matter and dark energy, we conclude that it is of interest to use the polarities of in modeling the causes of these conditions.

Indeed, given that positive polarity defines the matter (mass) verified in the galaxies, to balance the predicted sum in P 03, one can model the effect attributed to dark matter (at least in part) to an accumulation of negative polarity around galaxies. It is also possible to propose alternating layers of  polarized, forming gravitational belts.

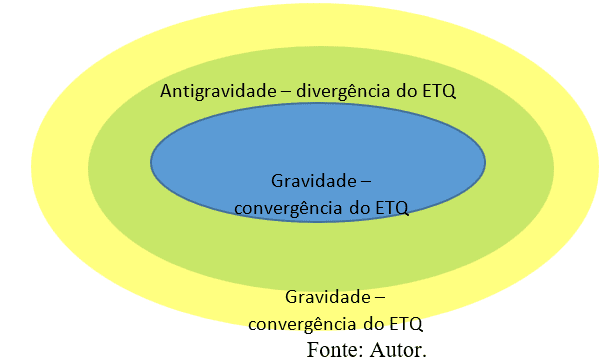
It is interesting to note that between the two regions of reversed polarities (the galaxy and dark matter) there will be antigravity in a null belt of polarities. Indeed, at the boundary of this belt with the galaxies, antigravitational forces press their periphery towards their interior, an effect of the ETQ flux from the divergence to the gradient. Then, as the modeling under construction is of the universe we observe, predictions can be made:

– Between a galaxy and the dark matter that surrounds it, there is at least a narrow region without relevant gravitational effects and, as a consequence, if there are more gravitational belts, they can also be observed through their effects, generating alternating regions with and without gravitational lensing ; and

– In the collision between galaxies, before the direct encounter of the matter that composes each one of them, the antigravitational effect will be perceptible given that the belt of   (dark matter) will be compressed further against the matter in the galaxies  .

So just as it spawns between the belts and the respective galaxies gravitational divergences (antigravitational effects attributed to dark energy – see Figure 02), in the space between galaxies, regions of alternating polarities of it’s from generate the effects attributed to dark energy. Note, then, that the explanation for the existence of regions with and without galaxies is given in terms of the existence of polarities in  .

Figure 02: illustration of the actions of gravity and antigravity in a galaxy (blue): the yellow and green belts indicate, respectively, domains of dark matter and energy.

It should be noted that the   subsets, when taken sequentially, constituting , result in an additional dimension (time) also defined by . In fact, we have Spaj in this temporal dimension identical to what occurs in any other of the dimensions (spatial).

For , that is, sufficiently close to the origin of the universe, the breaking of the superposition of states assigns polarities to the   coefficient, that is,  and, and clusters of these in an embryonic universe, defining the ETQ morphology and the disposition of the matter in it.

Similarly, the definition of natural laws verified in our universe is a particularity among the superimposed states of  , and there may even be a sequence in which neighbors are not correlated (chaos). It is, therefore, the relations of mutual induction between A and S, which occur along t, that define a particular  after loss of overlap.

It is interesting to note that the assumption of an ETQ allows for a solution to the following question.

According to General Relativity, for measured by 2 observers (X and Y) in reference frames with different strength gravitational fields, the interval   is measured larger by whoever is in the smaller gravitational field (assume it is X) in relation to the other observer (Y). In other words, the distance in the time dimension between the positions in   they do not coincide due to the different gravitational attractions of the frames of reference, exactly as in the spatial dimensions. It happens that, in space, when bodies do not collide, it is due to some opposite force or relative motion (such as planets in orbits): a meteor that approaches the Sun with a velocity lower than the escape velocity will inevitably collide with the star; and, considering black holes, when reaching the event horizon, it is not even possible to propose a realistic escape velocity. Two black holes can be far enough apart in space that there is no gravitational interaction between them. But in the space-time continuum, it is always possible to arbitrate    close as you like (including within the event horizon) and yet there is no (a strange) collapse in time of any massive bodies (stars, planets, a pan in the kitchen of a house or a proton in the nucleus of a a helium atom). In the continuous dimensional structure, there is no explanation for the non-existence of the aforementioned collapse.

The other forces found can be modeled with additional spatial dimensions and unit extension, as proposed by String Theory and its variants. But, unlike these, the proposal is that dimensions replace fields, like gravity. Indeed, take the example of the electromagnetic force.

Consider that k = 3 in equation (8) defines the actuation Spaj corresponding to the electromagnetic force. Although it is unitary in spatial extent, this is sufficient for a surface of unit thickness parallel to each spatial dimension and, as an effect, the attraction or repulsion in this parallel layer results in the acceleration of particles in the spatial dimension.

The difference between gravitational and electromagnetic Spaj is that for the latter we have:

– Identical polarities result in divergent, S3 generation; and

– Different polarities constitute gradients, contraction of S3.

Similar mechanisms can be applied to other forces (such as 3 unit dimensions for color charges, present in quarks and gluons) and other particle properties (spin, for example), provided that discrete modeling results in the continuous approximation presented in experiments and literature.

4. FINAL CONSIDERATIONS

In this article, a model was proposed for the unification of Quantum Mechanics and General Relativity: space, time and matter are treated as quantum information generated by a single physical variable. The proposal can either be used to generalize String Theory (and its variants), incorporating the ETQ, as well as to establish parameters that qualify particles and their relationship with discrete space-time, modifying the conception that messenger particles intermediate the relationship between the other particles for an intermediation performed by discrete dimensional units. In the latter case, in particular, the 3 expanded spatial dimensions of the ETQ constitute the gravitational field.

Additionally, the model provides an explanation for dark matter and dark energy, presenting gravitational and antigravity sources as a natural result of the parameters that define matter and the boundary conditions verified experimentally.

Finally, verifiable effects were proposed so that the model presented can be empirically tested.

The theory proposed in this study has gaps that prevent a complete explanation that describes our universe, such as: it is not able to explain the reason why the multiplicity of possible particles to be modeled, according to equation (10), is not observed; is not structured as a proposal that answers the reason why the observed dynamics, considering the k, j and t coordinates, specifically describes formula 83 of our universe, an issue apparently related to the question of the collapse of the wave function or measurements (BASSI et al., 2013), also not addressed in this study; does not provide a solution to the singular conditions of the beginning of the universe (PENROSE, 2006); and, although it makes it possible to model particularities of the evolution of the universe, such as Cosmic Inflation, it does not present an approach to possible causes.

As proposals for future work, in addition to experiments that can prove or disprove the present study, the deduction of discrete equations (or adequacy of existing ones) from the models available in the scientific literature on the subject is indicated and, from equation (10) , verify if there is a possible correlation with the Uncertainty Principle.

5. ACKNOWLEDGMENTS

For the attention and work they had, supporting me in correcting the text of this article, reviewing it and proposing suggestions, I would like to thank: Eduardo de Almeida Cadorin, Laís Santis de Oliveira, Marlanfe Michaelis Rocha de Oliveira, Michelena do Nascimento Santana, Paulo Cesar Pellanda and Saul de Oliveira Santana.

REFERENCES

ABDALLA, Elcio. Teoria quântica da gravitação: Cordas e Teoria M. Revista Brasileira de Ensino de Física, volume 27, número 1, pág. 147 – 155, 2005. Disponível em: https://www.scielo.br/j/rbef/a/sfYbTkSRk6ZFHBd6NPg7hqn/?format=pdf&lang=pt. Acesso em 26 de julho de 2021.

BARBÓN, J. L. F. String Theory. The European Physical Journal C – Particles and Fields volume 33, pagess67–s74, 2004. https://doi.org/10.1140/epjcd/s2003-03-009-5

BASSI, Angelo et al. Models of wave-function collapse, underlying theories, and experimental tests. Reviews of Modern Physics, v. 85, n. 2, p. 471, 2013. Disponível em: https://arxiv.org/pdf/1204.4325.pdf. Acesso em 04 de fevereiro de 2022.

BOJOWALD, Martin. Quantum cosmology: a review. Reports on Progress in Physics, Volume 78, Number 2, 12 de janeiro de 2015.

BURRUS, L. Sidney, GOPINATH, R. A. e GUO, Haitao. Introduction to Wavelets and Wavelet Transforms. Prentice Hall; 1ª edição, 268 páginas, 24 de agosto de 1997.

CHIAO, Raymond Y. Conceptual tensions between quantum mechanics and general relativity: Are there experimental consequences? Cambridge University Press, Wheeler’s 90th Birthday Symposium Proceedings, 2003. Disponível em: https://arxiv.org/pdf/gr-qc/0303100.pdf. Acesso em: 25 Jul 2021.

CORBELLI, Edvige. e SALUCCI, Paolo. The Extended Rotation Curve and the Dark Matter Halo of M33. Month. Notic. Royal Astron. Soc., V. 311, Issue 2, p. 441-447, 1999. DOI: 10.1046/j.1365-8711.2000.03075.x. Acesso em: 25 jul. 2021.

DIRAC, Paul Adrien Maurice. A new notation for quantum mechanics. Mathematical Proceedings of the Cambridge Philosophical Society , Volume 35 (3), Julho 1939 , pp. 416 – 418. https://doi.org/10.1017/S0305004100021162

DOPLICHER, Sergio; FREDENHAGEN, Klaus; e ROBERTS, John E. Spacetime quantization induced by classical gravity. Elsevier, Physics Letters B, Volume 331, Issues 1–2, Pages 39-44, 30 de junho de 1994. https://doi.org/10.1016/0370-2693(94)90940-7.

EINSTEIN, Albert. Relativity: The Special and the General Theory. Three Rivers Press, Illustrated edição (CA), 208 páginas, 06 de junho de 1995.

EINSTEIN, Albert. The General Theory of Relativity. In: The Meaning of Relativity. Springer, Dordrecht, 1922. Disponível em: https://doi.org/10.1007/978-94-011-6022-3_3. Acesso em 24/07/2021.

FENG, Jonathan L. Dark Matter Candidates from Particle Physics and Methods of Detection, Ann. Rev. Astron. Astrophys. v. 48, p. 495, 2010. DOI: 10.1146 / annurev-astro-082708-101659. Acesso em: 25 jul. 2021.

FEYNMAN, R. The character of physical law. The MIT Press, London, 10ª edição, 2017 (1ª edição, 1965).

GREENE, Brian. O Universo Elegante. Companhia das Letras, 1ª edição, 29 de março de 2001.

GUTH, Alan H.; STEINHARDT, Paul J. The inflationary universe. Scientific American, v. 250, n. 5, p. 116-129, 1984.

KAKU, Michio. Hiperespaço. Editora Rocco, 2000.

KUCHA, Karel. Gravity: Foundational Questions. SCIENCE, VOL. 239, 01 de janeiro de 1988. Disponível em: https://science.sciencemag.org/content/239/4835/80 Acesso em: 26 de julho de 2021.

MALLAT, Stéphane. A Wavelet Tour of Signal Processing. Elsevier, third ed. edition, 2009.

PENROSE, Roger. Before the big bang: an outrageous new perspective and its implications for particle physics. In: Proceedings of EPAC. 2006. p. 2759-2763. Disponível em: https://wyynd.tripod.com/rp.pdf. Acesso em 04 de fevereiro de 2022.

PIATTELLA, Oliver F. O artigo fundador da teoria da relatividade restrita: Sobre a eletrodinâmica dos corpos em movimento. Cadernos de Astronomia, Vitória, v. 1, n. 1, p. 157–176, 2020. DOI: 10.47083/Cad.Astro.v1n1.31681. Disponível em: https://periodicos.ufes.br/astronomia/article/view/31681. Acesso em 24/07/2021.

POLCHINSKI, Josepholchinski. Superstring Theory and Beyond, String Theory. Cambridge University Press, Volume 1, 1998.

RIESS, Adam G.; FILIPPENKO, Alexei V.; CHALLIS, Peter; CLOCCHIATTIA, Alejandro; DIERCKS, Alan; GARNAVICH, Peter M.; GILLILAND, Ron L.; HOGAN, Craig J.; JHA, Saurabh; KIRSHNER, Robert P.; LEIBUNDGUT, B.; PHILLIPS, M. M.; REISS, David; SCHMIDT, Brian P.; SCHOMMER, Robert A.; SMITH, R. Chris; SPYROMILIO, J.; STUBBS, Christopher; SUNTZEFF, Nicholas B.; e TONRY, John. Observational Evidence from Supernovae for an Accelerating Universe and a Cosmological Constant. Astron. Journ., volume 116, pág. 1009-1038, 1998. Disponível em: https://iopscience.iop.org/article/10.1086/300499/pdf. Acesso em: 25 jul. 2021.

SCHULZ, Benjamin. Review on the quantization of gravity. arXiv: 1409,7977 [gr-qc]. Disponível em: https://arxiv.org/pdf/1409.7977.pdf. Acesso em: 25 Jul 2021.

SMOLIN, Lee. Três Caminhos para a Gravidade Quântica. Editora Rocco, 2002.

SPINELLI, Miguel. A noção de arché no contexto da Filosofia dos Pré-Socráticos. Revista Hypnos, n. 8, 2002. Disponível em: https://hypnos.org.br/index.php/hypnos/article/view/124/126. Acesso em 04 de fevereiro de 2022.

STAMATESCU, Ion-Olimpiu e SEILER, Erhard (Eds.). Approaches to Fundamental Physics, Lect. Notes Phys. Springer, Berlin Heidelberg, número 721, 2007. DOI 10.1007/978-3-540-71117-9. Acesso em: 25 jul. 2021.

^[1] Doctor in Defense Engineering (focus on mechatronics), Master in Electrical Engineering (focus on control), specialist in Technological Innovation Management, graduated in Electrical Engineering and Military Sciences. ORCID: 0000-0002-9524-4643.

Sent: December, 2021.

Approved: February, 2022.